Ceramic Filters, April 1969 Electronics World

The introduction of low cost, small-footprint ceramic filters were unquestionably a boon to efforts at reduction in end-product package size and manufacturing cost. Very good Q and selectivity, no tuning required, and good temperature stability made them perfect for use as IF filters in broadcast radio receivers, at 10.7 MHz (FM) and 455 kHz (AM). They became available for commercial use around 1960 about a decade before this Electronics World magazine article hit the news stand. This publically available paper published in 2000 from the IEEE provides some historical perspective to ceramic filters: The History of Ceramic Filters, by Satoru Fujishima. The Clevite Corporation, for which author Reg Zimmerman worked, is mentioned in the IEEE paper, as is Murata, for being pioneers in the ceramic filter field.

Ceramic Filters

The author joined Clevite in 1958 as Sales Engineer for the Piezoelectric Division, subsequently moving to the Brush Instruments Division of the form. He rejoined the Piezo-electric Div. in 1965. He received his BEE from the University of Detroit. During World War II he served as Communications and Navigation Officer with the Navy in the Pacific.

Filters like these are a "must" for high-density packaging. An extremely high performance level and rugged construction boosts equipment efficiency.

The trend in military and commercial communications equipment for minimized components using IC integration has created a demand for extremely small filters that are rugged, reliable, and have relatively high sensitivity.

Filters made of ceramic, the newest material for the fabrication of bandpass filters, have these characteristics. For example, a filter with seventeen high-"Q" tuned circuits for bandwidth ratios of 1.1 to 1 from 70 dB to 6 dB attenuation can be packed in a 0.312-inch diameter tube less than 1.6 inches long.

The uniqueness of the ceramic filter results from the properties of the lead zirconate-lead titanate resonators. These resonators have a mechanical "Q" of 450 or 1600, depending on the particular composition. In addition, ceramic resonators are piezoelectric - by bending, squeezing, and twisting, the material directly converts mechanical movement to electrical energy. By reversing the process, it converts electrical energy into mechanical movement. The high electro-mechanical coupling provides wide-band filters with low insertion loss.

Typically, ceramic disks which measure about 0.2 inch in diameter by 0.010 to 0.040 inch thick resonate at 455 kHz.

Ceramic Filters: Ceramic filters have been developed to operate at frequencies from 40 Hz to 10.7 MHz. As was mentioned earlier, ceramic resonators have "Q's" of 450 and 1600. The higher "Q" materials make narrower bandwidth filters feasible. Higher "Q" materials also reduce insertion loss.

The stability of ceramic is 0.2% of the center frequency from -40°C to +85°C. This is equivalent to the best grade of LC filters, but not as good as mechanical or quartz filters. This can be offset by two design techniques. First, due to the steep sides of ceramic filters, the 6-dB bandwidth can be widened to allow for temperature change. Second, since the frequency change with temperature is a percentage of the center frequency, lowering the center frequency will reduce frequency shift. Thus, a 500-kHz ceramic filter has the same stability as a 15-MHz quartz filter.

Fig. 1 - These ceramic filters operate from 9 to 50 kHz and from 300 to 770 kHz. They are ideal for high-density packaging.

Fig. 2 - A group of resonators. (Left) resonates at 9 kHz, (center) at 167 kHz, and small one on the right at 455 kHz.

LC Filters: LC filters can be used over a wide frequency range, but their limiting factors are temperature stability (dependent on core material) and "Q". In general, "Q" (c.f.) / 3 dB (bandwidth) is less than 100 for LC filters. Powdered iron cores have good stability but low permeability, lowering the "Q" of the inductor. Ferrite cores, on the other hand, offer higher permeability but at the expense of temperature stability. Also, low-frequency filters require large cores, thus increasing weight and size. One more point, the low "Q" in LC filters produces a filter with a higher insertion loss when sections are cascaded to steepen the sides of the passband.

Mechanical Filters: Mechanical filters have a "Q" of 7000 and excellent temperature stability. These properties favor narrow-band filters (less than 1%) such as single-sideband filters. However, the mechanical filter requires a transducer to convert electrical energy to mechanical energy and another transducer to reconvert the mechanical energy to electrical energy. This, in turn, increases insertion loss.

Quartz Filters: Quartz filters have "Q's" ranging from 50,000 to 100,000. Their temperature stability is excellent, but they are generally more expensive than LC or ceramic filters. Bandwidths are usually limited to less than 1% of center frequency unless broadbanding inductors are used. However, competitively priced products are available at 10.7 MHz.

Currently, three resonator designs are used in manufacturing the filters shown in Fig. 1. The split ring shown in Fig. 2 resonates at 9 kHz but it can be tuned to 50 kHz by widening the slot. This thin disk, which has an inductance value equivalent to about 50 henrys, vibrates at its resonant frequency by the expansion and contraction of the slot.

The center thin disk vibrates radially at its overtone frequency, popularly 455 kHz. If operated in its fundamental mode, it would vibrate at 167 kHz. Differences in the electrode area on a given disk result in an impedance transformation so that the filters of this type can replace the common i.f. transformer, yielding higher "Q", smaller size, greater temperature stability, and greater selectivity.

The small-sized disk (at right) is the basic resonator used for oscillator control and for ladder filters. It utilizes the radial mode of vibration, i.e., it expands and contracts radially. Its resonant frequency is determined by its diameter.

Fig. 3 - Circuit and performance curve of ceramic resonator.

Fig. 4 - Two-resonator circuit arid its electrical equivalent.

A fully electroded disk has an overtone at 2.3 times its fundamental frequency which can be suppressed or enhanced by varying the area the electrode covers. The center of the disk is a vibrational node point. Electrical connections are made by pressure contacts at the center of the disk. The equivalent circuit and the curve of impedance versus frequency are shown in Fig. 3.

In single-resonator circuits, the equation for the 3-dB bandwidth point is B = f_o / Q_L, where f_o is the center frequency and Q_L is the loaded "Q".

Single resonators can be used to control oscillator frequencies or to replace emitter-bypass capacitors and sharpen the frequency response of transistor radio receivers.

Generally, two or more resonators are required to develop the desired bandpass characteristics. The basic two-resonator circuit and its electrical equivalent circuit are shown in Fig. 4.

The two-resonator section is referred to as an L-section. Assuming 455-kHz is the desired f_o, by tuning the 10K-impedance point (f_r) of the series resonator and the high impedance (f_a) of the shunt resonator to f_o, the minimum signal attenuation will be at f_o. Likewise, the points of maximum signal attenuation will be at f_a of the series resonator and f_r of the shunt resonator. If resonators with the same Δf are used, the attenuation curve illustrated in Fig. 5 will result.

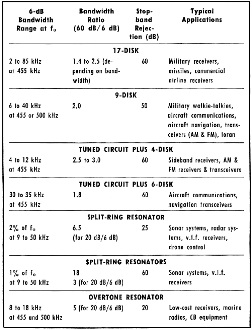
Table 1. Specifications and applications of resonators.

The C_o of the individual resonator is determined by the electroded area, the dielectric constant, and the disk thickness. At frequencies above and below the high attenuation poles, both resonators act like a capacitance divider. The larger the ratio C_shunt / C_series, the larger the attenuation in the stopband. Since the resonator "Q", f_r, Δf, and C_o are all variable in the manufacturing process, center frequency, the passband width, and the attenuation pole locations can be varied simply and inexpensively.

The nine-disk and seventeen-disk ladder filters are electrically connected L-sections with an extra series resonator at the end to make the input and output impedances equal. Since ceramic filters have their maximum attenuation just above and below the passband, they offer maximum rejection to adjacent signals. Most LC and quartz filters have their maximum attenuation points at frequencies of zero and infinity. Therefore, ceramic filters in the second i.f. of double-conversion receivers complement the attenuation pattern of the r.f. stage and the first i.f. and greatly improve the receiver's characteristics. Ceramic ladder filters have stop-band attenuations from 50 dB to 90 d B, depending on the model. The stop bands are free of spurious responses from d.c. to 2.3 times the passband frequency.

Ladder filters are simple to use. They are especially designed to match resistive loads of 1000 to 2500 ohms, depending on the model; and are suitable for use with transistorized circuitry (see Fig. 6).

Response curve of the two-resonator circuit - RF Cafe

Fig. 5 - Response curve of the two-resonator circuit shown in Fig. 4.

Fig. 6 - Ladder filters are designed to match the resistive loads of 1000 to 2500 ohms found in transistorized circuits.

Fig. 7 - 60-dB stopband in a narrow-band filter can be achieved when a tuned LC circuit is combined with four resonators.

When calling out ceramic filter parameters, the design engineer should specify minimum stopband attenuation, maximum insertion loss, and maximum passband ripple as with other filters types. He should, however, also call out the minimum 6-dB and the maximum 60-dB bandwidths.

Generally, filter impedance level is set by the manufacturer and is a function of center frequency and bandwidth and hence cannot be specified by the designer.

The designer should be aware that the filter's center frequency and bandwidth changes over the operating temperature range. For instance, center frequency of a 500-kHz filter will change a maximum of 1 kHz (0.2%) over the temperature range -40° C and +85° C. The 6-dB bandwidth is usually designed so that over this temperature range it stays larger than the minimum specification. In the frequency range 300 kHz to 700 kHz, the 6-dB bandwidth achievable with ceramic filters range from approximately 0.5% of center frequency to 20% of center frequency.

One of the lowest-cost filters designed by Clevite combines a tuned LC circuit with resonators having the maximum capacitance divider ratio. Thus, four resonators in a narrow-band filter design achieve 60-dB stopband attenuation; six resonators are required for wideband filters. The schematic of this low-cost unit is shown in Fig. 7 and Table 1 lists its specifications and applications as well as those of some other typical units.

Because ceramic filters are small in size, very rugged, fixed tuned, and extremely reliable, their future is bright.

Ceramic filters are competitively priced and are rapidly making inroads in low-cost entertainment equipment. They are especially attractive since they do not require the installation alignment of conventional tuned circuits.

Ceramic filters have already replaced larger, more expensive LC and mechanical filters in many mobile, portable, and Citizens Band receivers. They have also repeatedly proven themselves in military equipment as the most selective and rugged filters for their size and weight.

Ceramic FiltersApril 1969 Electronics World

Ceramic Filters

Ceramic Filters
April 1969 Electronics World