 Ask and ye shall receive...
at least sometimes. I posted a request for an article by science fiction
writer Arthur C. Clarke,
of 2001: A Space Odyssey fame, describing a geostationary satellite system that was published
in the October 1945 edition of Wireless World magazine. Thanks to RF Cafe visitor
Terry W., from the great state of Oklahoma, it is now available for everyone to
enjoy. Clarke was not just a sci-fi writer, but also an educated visionary and card-carrying
member of the British Interplanetary Society, who
proposed many technological solutions to issues of his day. In this instance, the
challenge was developing an efficient means to distribute TV signals across Europe
and the world. Clarke's calculations for the necessary number of repeater towers
proved that concept impractical, so he proposed using modified surplus German V2
rockets to launch Earth-orbiting "artificial satellites," powered by batteries or
solar panels, to distribute signals globally. He calculated power densities, orbit
altitudes and periods, S/N ratios, and antenna sizes. You will want to read this
article; it only takes about 15-20 minutes.
See also
Peacetime Uses for V2
Can Rocket Stations Give World-Wide Radio Coverage?
 By Arthur C. Clarke By Arthur C. Clarke
Although it is possible, by a suitable choice of frequencies and routes,
to provide telephony circuits between any two points or regions of the earth for
a large part of the time, long-distance communication is greatly hampered by the
peculiarities or the ionosphere, and there are even occasions when it may be impossible.
A true broadcast service, giving constant field strength at all times over the whole
globe would be invaluable, not to say indispensable, in a world society.
Unsatisfactory though the telephony and
telegraph position is, that of television is far worse, since ionospheric transmission
cannot be employed at all. The service area of a television station, even on a very
good site, is only about a hundred miles across. To cover a small country such as
Great Britain would require a network of transmitters, connected by coaxial lines,
waveguides or VHF relay links. A recent theoretical study1 has shown
that such a system would require repeaters at intervals of fifty miles or less.
A system of that kind could provide television coverage, at a very considerable
cost, over the whole of a small country. It would be out of .the question to provide
a large continent with such a service, and only the main centres of population could
be included in the network.
The problem is equally serious when an attempt is made to link television services
in different parts of the globe. A relay chain several thousand miles long would
cost millions, and transoceanic services would still be impossible. Similar considerations
apply to the provision of wide-band frequency modulation and other services, such
as high-speed facsimile which are by their nature restricted to the ultra-high-frequencies.
Many may consider the solution proposed in this discussion too far-fetched to
be taken seriously. Such an attitude is unreasonable, as everything envisaged here
is a logical extension of developments in the last ten years - in particular the
perfection of the long-range rocket of which
V2 was the prototype. While this
article was being written, it was announced that the Germans were considering a
similar project, which they believed possible within fifty to a hundred years.
Before proceeding further, it is necessary to discuss briefly certain fundamental
laws of rocket propulsion and "astronautics." A rocket which achieved a. sufficiently
great speed in flight outside the earth's atmosphere would never return. This "orbital"
velocity is 8 km per sec, (5 miles per sec), and a rocket which attained it would
become an artificial satellite, circling the world forever with no expenditure of
power - a second moon, in fact. The German transatlantic rocket Aro to would. have
reached more than half this velocity.
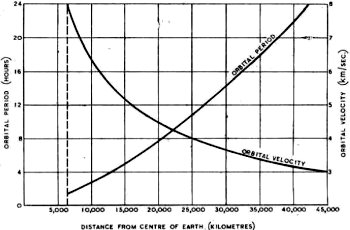
Fig. 1 - Variation of orbital period and velocity
with distance from the centre of the earth.
It will be possible in a few more years to build radio controlled rockets which
can be steered into such orbits beyond the limits of the atmosphere and left to
broadcast scientific information back to the earth. A little later, manned rockets
will be able to make similar flights with sufficient excess power to break the orbit
and return to earth.
There are an infinite number of possible stable orbits, circular and elliptical,
in which a rocket would remain if the initial conditions were correct. The velocity
of 8 km/sec. applies only to the closest possible orbit, one just outside the atmosphere,
and the period of revolution would be about 90 minutes. As the radius of the orbit
increases the velocity decreases, since gravity is diminishing and less centrifugal
force is needed to balance it. Fig. 1 shows this graphically. The moon, of course,
is a particular case and would lie on the curves of Fig. 1 if they were produced.
The proposed German space-stations would have a period of about four and a half
hours. It will be observed that one orbit, with a radius of 42,000 km, has a period
of exactly 24 hours. A body in such an orbit, if its plane coincided with that of
the earth's equator, would revolve with the earth and would thus be stationary above
the same spot on the planet. It would remain fixed in the sky of a whole hemisphere
and unlike all other heavenly bodies would neither rise nor set. A body in a smaller
orbit would revolve more quickly than the earth and so would rise in the west, as
indeed happens with the inner moon of Mars.
Using material ferried up by rockets, it would be possible to construct a "space-station"
in such an orbit. The station could be provided with living quarters, laboratories
and everything needed for the comfort of its crew, who would be relieved and provisioned
by a regular rocket service. This project might be undertaken for purely scientific
reasons as it would contribute enormously to our knowledge of astronomy, physics
and meteorology. A good deal of literature has already been written on the subject.2
Although such an undertaking may seem Fantastic, it requires for its fulfillment
rockets only twice as fast as those already in the design stage. Since the gravitational
stresses involved in the structure are negligible, only the very lightest materials
would be necessary and the station could be as large as required.

Fig. 2 - Typical extra-terrestrial relay services.
Transmission from A being relayed to points B and area C; transmission from D
being relayed to whole hemisphere.
Let us now suppose that such a station were built in this orbit. It could be
provided with receiving and transmitting equipment (the problem of power will be
discussed later) and could act as a repeater to relay transmissions between any
two points on the hemisphere beneath, using any frequency which will penetrate the
ionosphere. If directive arrays were used, the power requirements would be very
small, as direct line of sight transmission would be used, There is the further
important point that arrays on the earth, once set up, could remain fixed indefinitely.
Moreover, a transmission received. from any point on the hemisphere could be
broadcast to the whole of the visible face of the globe, and thus the requirements
of all possible services would be met (Fig. 2)
It may be argued that we have as yet no direct evidence of radio waves passing
between the surface of the earth and outer space; all we can say with certainty
is that the shorter wavelengths are not reflected back to the earth. Direct evidence
of field strength above the earth's atmosphere could be obtained by V2 rocket technique,
and it is to be hoped that someone will do something about this soon as there must
be quite a surplus stock somewhere! Alternatively, given sufficient transmitting
power, we might obtain the necessary evidence by exploring for echoes from the moon.
In the meantime we have visual evidence that frequencies at the optical end of the
spectrum pass through with little absorption except at certain frequencies at which
resonance effects occur. Medium high frequencies go through the E layer twice to
be reflected from the F layer and echoes have been received from meteors in or above
the F layer. It seems fairly certain that frequencies from, say, 50 Mc/s to 100,000
Mc/s could be used without undue absorption in the atmosphere or the ionosphere.
A single station could only provide coverage to half the globe, and for a world
service three would be required, though more could be readily utilized. Fig. 3 shows
the simplest arrangement. The stations would be arranged approximately equidistantly
around the earth, and the following longitudes appear to be suitable:-
30 E - Africa. and Europe. 150 E - China
and Oceana. 90 W - The Americas.
The stations in the chain would be linked by radio or optical beams, and thus
any conceivable beam or broadcast service could be provided.

Fig. 3 - Three satellite stations would ensure
complete coverage of the globe.
The technical problems involved in the design of such stations are extremely
interesting,3 but only a few can be gone into here, Batteries of parabolic
reflectors would be provided, of apertures depending on the frequencies employed.
Assuming the use of 3,000 Mc/s waves, mirrors about a metre across would beam almost
all the power on to the earth. Larger reflectors could be used to illuminate single
countries or regions for the more restricted services, with consequent economy of
.power. On the higher frequencies it is not difficult to produce beams less than
a degree in width, and, as mentioned before, there would be no physical limitations
on the size of the mirrors. (From the space station, the disc of the earth would
be a little over 17 degrees across). The same mirrors could be used for many different
transmissions if precautions were taken to avoid cross modulation.
It is dear from the nature of the system that the power needed will be much less
than that required for any other arrangement, since all the energy radiated can
be uniformly distributed over the service area, and none is wasted. An approximate
estimate of the power required for the broadcast service from a single station can
be made as follows;-
The field strength in the equatorial plane of a λ/2 dipole in free space at a
distance of d metres is4
e = 6.85 √P / d volts/metre, where P is
the power radiated in watts.
Taking d as 42,000 km (effectively it would be less), we have
P=37.6 e2 watts. (e now in μV/metre.)
If we assume e to be 50 microvolts/metre, which is the F.C.C. standard for frequency
modulation, P will be 94 kw. This is the power required for a single dipole, and
not an array which would concentrate all the power on the earth, Such an array would
have a gain over a simple dipole of about 80. The power required for the broadcast
service would thus be about 1.2 kW.

A project which goes part of the way towards the goal envisaged
in this article has been put forward by Westinghouse in collaboration with the
Glen L. Martin Co. of America. The radius of coverage would be increased from
50 to 211 miles by beamed radiation from an aircraft flying at a height of 30,000
ft., and equipped with television and FM transmitters.
Ridiculously small though it is, this figure is probably much too generous. Small
parabolas about a foot in diameter would be used for receiving at the earth end
and would give a very good signal/noise ratio. There would be very little interference,
partly because of the frequency used and partly because the mirrors would be pointing
towards the sky which could contain no other source of signal. A field strength
of 10 microvolts/metre might well be ample, and this would require a transmitter
output of only 50 watts.
When it is remembered that these figures relate to the broadcast service, the
efficiency of the system will be realised. The pointto-point beam transmissions
might need powers of only 10 watts or so. These figures, of course, would need correction
for ionospheric and atmospheric absorption, but that would be quite small over most
of the band. The slight falling off in field strength due to this cause towards
the edge of the service area could be readily corrected by a nonuniform radiator.
The efficiency of the system is strikingly revealed when we consider that the
London Television service required about 3 kW average power for an area less than
fifty miles in radius.5
A second fundamental problem is the provision of electrical energy to run the
large number of transmitters required for the different services. In space beyond
the atmosphere, a square metre normal to the solar radiation intercepts 1.35 kW
of energy.6 Solar engines have already been devised for terrestrial use
and are an economic proposition in tropical countries. They employ mirrors to concentrate
sunlight on the boiler of a low-pressure steam engine. Although this arrangement
is not very efficient it could be made much more so in space where the operating
components are in a vacuum, the radiation is intense and continuous, and the low-temperature
end of the cycle could be not far from absolute zero. Thermoelectric and photoelectric
developments may make it possible to utilise the solar energy more directly.

Fig. 4 - Solar radiation would be cut off for
a short period each day at the equinoxes.
Though there is no limit to the size of the mirrors that could be built, one
fifty metres in radius would intercept over 10,000 kW and at least a quarter of
this energy should be available for use.
The station would be in continuous sunlight except for some weeks around the
equinoxes, when it would enter the earth's shadow for a few minutes every day. Fig.-4
shows the state of affairs during the eclipse period. For this calculation, it is
legitimate to consider the earth as fixed and the sun as moving round it. The station
would graze the earth's shadow at A, on the last day in February. Every day, as
it made its diurnal revolution, it would cut more deeply into the shadow, undergoing
its period of maximum eclipse on March 21st, on that day it would only be in darkness
for 1 hour 9 minutes From then onwards the period of eclipse would shorten, and
after April 11th (B) the station would be in continuous sunlight again until the
same thing happened six months later at the autumn equinox, between September 12th
and October 14th The total period of darkness would be about two days per year,
and as the longest period of eclipse would be little more than an hour there should
be no difficulty in storing enough power for an uninterrupted service.
Conclusion
Briefly summarised , the advantages of the space station are as follows:-
(1) It is the only way in which true world coverage call be achieved for all
possible types of service. (2) It permits unrestricted use of a band at least
100,000 Mc/s wide, and with the use of beams an almost unlimited number of channels
would be available. (3) The power requirements are extremely smart since the
efficiency of "illumination" will be almost 100 per cent. Moreover, the cost of
the power would be very low. (4) However great the initial expense, it would
only be a fraction of that required for the world networks replaced, and the running
costs would be incomparably less.
Appendix - Rocket Design
The development of rockets sufficiently powerful to reach "orbital" and even "escape"
velocity is now only a matter of years. The following figures may be of interest
in this connection.
The rocket has to acquire a final velocity of 8 km/sec. Allowing 2 km/sec for
navigational corrections and air resistance less (this is legitimate as all space-rockets
will be launched from very high country) gives a total velocity needed of 10 km/sec.
The fundamental equation of rocket motion is:2
V = v loge R
where V is the final velocity of the rocket, v the exhaust velocity and R the
ratio of initial mass to final mass (payload plus structure). So far v has been
about 2-2.5 km/sec for liquid fuel rockets but new designs and fuels will permit
of considerably higher figures, (Oxyhydrogen fuel has a theoretical exhaust velocity
of 5.2 km/sec and more powerful combinations are known). If we assume v to be 3.3
km/sec, R will be 20 to 1. However, owing to its finite acceleration, the rocket
loses velocity as a result of gravitational retardation. If its acceleration (assumed
constant) is α meters/sec2, then the necessary ratio Rg is
increased to
Rg = R (α +g) / α
For an automatically controlled rocket α would be about 5g and so the necessary
R would be 37 to 1. Such ratios cannot be realised with a single rocket but can
be attained by "step-rockets"2, while very much higher ratios (up to
1,000 to 1) can be achieved by the principle of "cellular construction."3
Epilogue - Atomic Power
The advent of atomic power has at one bound brought space travel half a century
nearer. It seems unlikely that we will have to wait much as twenty years before
atomic-powered rockets are developed. and such rockets could reach even the remoter
planets with a fantastically small fuel/mass ratio ---only a few per cent. The equations
developed in the appendix still hold, but v will be increased by a factor of about.
a thousand.
In view of these facts, it appears hardly worth, while to expend much effort
on the building of long-distance relay chains. Even the local networks which will
soon be under construction may have a working life of only 20-30 years.
References
1 Radio-Relay Systems," C. W. Hansell. Proc. I.R.E.,. Vol 33, March
1945 2 "Rockets," Willy Ley. (Viking Press, N.Y.) 3
"Das Problem der Befahrung des Weltraums," Hermann Noordung. 4
"Frequency Modulation," A. Hund. (McGraw Hill). 5 "London Television
Service," MacNamara amd Birkinshaw. J.I.E.E., Dec. 1938. 6
"The Sun," C. G. Abbot. (Appleton-Century Co.). 7 "Journal
of the British Interplanetary Society," Jan., 1939.
Posted February 22, 2019
(updated from original post on 7/5/2011)
|

































