June 1944 QST
 Table
of Contents Table
of Contents
Wax nostalgic about and learn from the history of early electronics. See articles
from
QST, published December 1915 - present (visit ARRL
for info). All copyrights hereby acknowledged.
|
Recognizing that many people were reluctant
to approach the theoretical aspect of electronics as it applied to circuit design
and analysis, QST (the American Radio Relay League's monthly publication)
included equations and explanations in many of their project building articles.
Occasionally, an article would be published that dealt specifically with how to
use simple mathematics. In this case, the June 1944 edition, we have the second
installation of at least a four-part tutorial that covers resistance and reactance,
amplifier biasing (tubes since the Shockley-Bardeen-Brattain trio hadn't invented
the transistor yet) oscillators, feedback circuits, etc. I do not have Part I
from the
May 1944
edition or Part IV from the
August
1944 edition, but if you want to send me those editions, I'll be glad to scan
and post them (see
Part
III here).
Practical Applications of Simple Math: Part II - Plate and Screen
Voltages
By Edward M. Noll, EX-W3FQJ
Whenever the d.c. plate current flows through any resistance placed in the plate
circuit of a vacuum tube as a load or coupling medium, it is obvious that the voltage
at the plate will be less than the supply voltage because of the voltage drop across
the resistance.

In Fig. 1 the plate voltage is
Ep = Eb - RpIp.
Example: In Fig. 1,
Eb = 250 volts. Rp = 10,000 ohms.
Ip = 10 ma. (0.01 amp.).
What is the plate voltage, Ep?
Ep = 250 - (10,000) (0.01) = 250 - 100 = 150 volts.
Since true plate voltage is the voltage between plate and cathode, the voltage
drop across the cathode resistor, Rk in Fig. 2, as well as the drop
across the plate resistor, Rp, must be subtracted from the supply voltage
in calculating plate voltage.
 In Fig. 2 the plate voltage is In Fig. 2 the plate voltage is
Ep = Eb - IpRp- IpRk.
= Eb - Ip(Rp + Rk).
Example: In Fig. 2,
Eb = 250 volts.
Rp = 25,000 ohms.
Rk = 2000 ohms.
Ip = 5 ma. (0.005 amp.).
What is the plate voltage, Ep?
Ep = 250 - (0. 005) (25,000 + 2000)
= 250 - (0.005) (27,000) = 250 - 135
= 115 volts.
One advantage of transformer coupling between audio-amplifier stages is that
the inductance of the transformer primary winding will provide a high-impedance
load for the tube at audio frequencies, while the d.c. resistance of the winding
is sufficiently low to cause only a small drop in d.c. plate voltage.
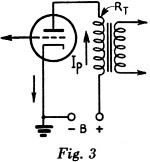 In Fig. 3 the only resistance affecting
the plate voltage is that of the transformer primary winding, Rt, so In Fig. 3 the only resistance affecting
the plate voltage is that of the transformer primary winding, Rt, so
Ep = Eb - IpRt
Example: In Fig. 3,
Ep = 250 volts. Ip = 20 ma. (0.02 amp.)
Rt = 100 ohms.
What is the plate voltage, Ep?
Ep = 250 - (0.02) (100) = 250 - 2
= 248 volts.
Screen voltage is determined in the same manner as plate voltage, using the screen
current to calculate the voltage drop across the screen resistor.
Ep = Eb - IpRp
Es = Eb - IsRs
 Example: In Fig. 4, Example: In Fig. 4,
Eb = 250 volts. Ip = 5 ma. (0.005 amp.).
Rp = 20,000 ohms. Is = 2 ma. (0.002 amp.).
Rs = 75,000 ohms.
What are the plate voltage, Ep, and screen voltage, Es?
Ep = 250 - (0.005) (20,000) = 250 - 100 = 150 volts.
Es = 250 - (0.002) (75,000) = 250 - 150 = 100 volts.
In the circuit of Fig. 5, both plate and screen currents flow through the
common resistor, R1, so that plate and screen currents must be added
in calculating the voltage drop across R1.
Ep = Eb - (Ip + Is) (R1)
- IpRp
Es = Eb -" (Ip + Is) (R1)
- IsRs.
 Example: In Fig. 5, Example: In Fig. 5,
Eb = 250 volts. Rp = 40,000 ohms.
Rs = 200,000 ohms. Ip = 2 ma, (0.002 amp.)
Is = 0.5ma. (0.0005 amp.). R1 = 20,000 ohms.
What are the plate voltage, Ep, and screen voltage, Es?
Ep = 250 - (0.002 + 0.0005) (20,000) - (0.002) (40,000)
= 250 - 50 - 80
= 120 volts.
Es= 250 - 50- (0. 0005) (200,000)
= 250 - 50 - 100
= 100 volts.
 In the circuit of Fig. 6-A, the screen
voltage, Es, is obtained from a tap on a voltage divider consisting of
Rs and Rb The equivalent circuit is shown in Fig. 6-B.
The screen voltage, Es, is equal to the voltage drop across Rb.
Therefore, In the circuit of Fig. 6-A, the screen
voltage, Es, is obtained from a tap on a voltage divider consisting of
Rs and Rb The equivalent circuit is shown in Fig. 6-B.
The screen voltage, Es, is equal to the voltage drop across Rb.
Therefore,
Es = RbIb.
Example: In Fig. 6-B,
Eb = 250. Is = 1 ma. Rs = 10,000 ohms.
Rb = 40,000 ohms.
What is the screen voltage, Es?
Es = IbRb.
Since Eb is equal to the sum of the voltages across Rs
and Rb,
Eb = RsIsr + RbIb.
Also, since both Ib and Is must flow through Rs,
Isr = Ib + Is.
Substituting this value for Isr in the above equation,
Eb = Rs (Ib + Is) + RbIb.
Transposing,
RsIb + RbIb = Eb - RsIs
Ib(Rs + Rb) = Eb - RsIs

Substituting known values,

Then,
Es = (0.0048) (40,000) = 192 volts.
 In the circuit of Fig. 7, both screen and
grid-biasing voltages are taken from voltage dividers. In the case of the divider
in the grid circuit, the voltage division is in exact proportion to the resistance
values of the divider sections, since it is assumed that the grid is biased so that
no grid current flows. Therefore, the grid-biasing voltage, Eg, is the
voltage developed across R2 by virtue of the current flowing through
it from the bias supply. In the circuit of Fig. 7, both screen and
grid-biasing voltages are taken from voltage dividers. In the case of the divider
in the grid circuit, the voltage division is in exact proportion to the resistance
values of the divider sections, since it is assumed that the grid is biased so that
no grid current flows. Therefore, the grid-biasing voltage, Eg, is the
voltage developed across R2 by virtue of the current flowing through
it from the bias supply.
Eg = IgR2
 being the bias-supply
voltage. being the bias-supply
voltage.
Substituting, 
Screen and plate voltages are calculated as before.
Example: In Fig. 7,
Eb = 250 volts. Ec = 100 volts.
R1 = 49,000 ohms.
R2 = 1000 ohms. R3 = 30,000 ohms.
R4 = 20,000 ohms. R5 = 20,000 ohms.
Is = 1 ma. (0.001 amp.).
Ip = 5 ma. (0.005 amp.).
What are the grid-biasing, screen and plate voltages?
Eg = 
= 2 volts (negative in respect to cathode).
Ep = 250 - (0.005) (20,000) = 250 - 100
= 1.50 volts.
Es = IbR3

= 0.0046 amp.
Es = (0.0046) (30,000) = 138 volts.
 Fig. 8 is used to illustrate the effects
of low voltmeter resistance upon the accuracy of voltage measurements. Rm
is the meter resistance. Fig. 8 is used to illustrate the effects
of low voltmeter resistance upon the accuracy of voltage measurements. Rm
is the meter resistance.
With the meter disconnected, the plate voltage will be
Ep = Eb - RpIp.
However, with the meter connected, the current, Im, will flow through
Rp. Thus, the voltage drop across Rp will increase and the
plate voltage will be lowered, especially when the resistance of the meter is low
in comparison with Rp. The equivalent circuit with the meter connected
is shown in Fig. 8-B, in which Rpi is the internal resistance of
the tube which is assumed to be constant with a change in plate voltage. The new
plate voltage desired is the voltage across Rpi (or Rm) which
is
Ep = Eb - (Ipm) (Rp),
where Ipm is the new current when Rm is connected. In other
words, Ep is the difference between the terminal voltage and the voltage
drop across Rp.
Example: In Fig. 8,
Eb = 250 volts. Ipi =0.1 ma. (0.0001 amp.)
Rp = 1 megohm (1,000,000 ohms)
Rm = 1000 ohms per volt (300-volt scale).
What is the true plate voltage with the meter disconnected and what voltage will
be measured by the meter when it is connected?
Ep = 250 - (1,000,000) (0.0001)
= 250 - 100
= 1.50 volts = plate voltage without meter connected.
As stated above, when the meter is connected,
Ep - Eb - (Ipm) (Rp).
Since Ipm is not known, its value must be found before the equation
can be solved. To find Ipm, the resultant resistance of Rm
and Rpi in parallel must be found, and this, in turn, requires that Rpi
be determined. This can be done by considering the circuit before the meter is connected.
The total circuit resistance, Rt is then given by
 = 2,500,000 ohms = 2,500,000 ohms
= 2.5 megohms.
Also,
Rt = Rp + Rpi
Rpi = Rt - Rp = 2,500,000 - 1,000,000
Rpi = 1,500,000 ohms = 1.5 megohms.
The resistance of the meter, Rm, is given as 1000 ohms per volt. Since
the meter has a 300-volt scale, its resistance is 300,000 ohms, or 0.3 megohm.
Rpim, the resultant resistance of Rpi and Rm
in parallel is given by
Rpim = 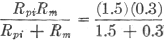
This gives the total circuit resistance in Fig. 8-B as
Rt = Rp + Rpim = 1 + 0.25 + 1.25 megohms.
The new current, Ipm, is then
Ipm = 
Then
Ep = Eb - (Ipm) (Rp)
= 250 - (0.0002) (1,000,000)
= 250 - 200 = 50 volts = voltage indicated by meter
reading.
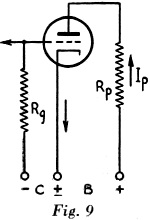 Example: In the case of Fig. 9,
it is assumed that the grid is to be fed a square-wave pulse. Compare the plate
voltage when the tube is conducting a current of 15 ma. with the effective plate
voltage when the tube is idle and not drawing plate current. The plate resistance
is 10,000 ohms. Example: In the case of Fig. 9,
it is assumed that the grid is to be fed a square-wave pulse. Compare the plate
voltage when the tube is conducting a current of 15 ma. with the effective plate
voltage when the tube is idle and not drawing plate current. The plate resistance
is 10,000 ohms.
When the tube is conducting,
Ep = Eb - IpRp ;= 250- (0.015) (10,000)
= 250 - 150 = 100 volts.
When the tube is not conducting, there is no voltage drop across Rp
and the plate voltage is 250, the same as the supply voltage, Eb.
 Fig. 10 illustrates another
use for the voltages divider. The coupling circuit shown is that commonly found
in direct-coupled amplifiers. From the equivalent circuit of Fig. 10-B, it
will be seem that the plate of the first tube is connected at one tap on the voltage
divider, while the grid is connected at another tap less positive. It is assumed
that the grid of the second tube is biased, by the voltage drop across its cathode
resistor, so that the grid does not draw current. Fig. 10 illustrates another
use for the voltages divider. The coupling circuit shown is that commonly found
in direct-coupled amplifiers. From the equivalent circuit of Fig. 10-B, it
will be seem that the plate of the first tube is connected at one tap on the voltage
divider, while the grid is connected at another tap less positive. It is assumed
that the grid of the second tube is biased, by the voltage drop across its cathode
resistor, so that the grid does not draw current.
Example: In Fig. 10,
Eb = 250 volts. Ip = 5 ma. (0.005 amp.)
R1 = 10,000 ohms. R2 = 75,000 ohms
R3 = 25,000 ohms.
What are the plate voltage of the first tube, and the grid voltage of the second
tube?
The total drop across all resistors is, of course, equal to the applied voltage,
Eb. The voltage, across R1 is R1I1,
while that across R2 and R3 in series is (R2 +
R3) (I2), bearing in mind that no current is being drawn from
the tap, marked G in Fig. 10-B, so that the same current flows through. R2
and R3. Then,
Eb = R1I1+ (R2 + R3) (I2)
Since both Ip and I2 flow through R1,
I1 = Ip + I2
Substituting this value for I1 in the preceding equation,
Eb = (Ip + I2) (R1) + (R2
+ R3) (I2).
Substituting known values,
250 = (0.005 + I2) (10,000) + (75,000 + 25,000) (I2)
= 10,000I2 + 50 + 100,000I2
110,000I2 = 200
I2= 0.0018 amp. = 1.8 ma.
The plate voltage of the first tube is equal to the sum of the voltage drops
across R2 and R3.
Ep = I2 (R2 + R3) = (0.0018) (100,000)
= 180 volts.
The grid voltage of the second tube is equal, to the voltage drop across R3.
Eg = I2R3= (0.0018) (25,000) = 45 volts.
|









 In Fig. 2 the plate voltage is
In Fig. 2 the plate voltage is  In Fig. 3 the only resistance affecting
the plate voltage is that of the transformer primary winding, Rt, so
In Fig. 3 the only resistance affecting
the plate voltage is that of the transformer primary winding, Rt, so
 Example: In Fig. 4,
Example: In Fig. 4,
 Example: In Fig. 5,
Example: In Fig. 5,



 In the circuit of Fig. 7, both screen and
grid-biasing voltages are taken from voltage dividers. In the case of the divider
in the grid circuit, the voltage division is in exact proportion to the resistance
values of the divider sections, since it is assumed that the grid is biased so that
no grid current flows. Therefore, the grid-biasing voltage, Eg, is the
voltage developed across R2 by virtue of the current flowing through
it from the bias supply.
In the circuit of Fig. 7, both screen and
grid-biasing voltages are taken from voltage dividers. In the case of the divider
in the grid circuit, the voltage division is in exact proportion to the resistance
values of the divider sections, since it is assumed that the grid is biased so that
no grid current flows. Therefore, the grid-biasing voltage, Eg, is the
voltage developed across R2 by virtue of the current flowing through
it from the bias supply.  being the bias-supply
voltage.
being the bias-supply
voltage. 



 = 2,500,000 ohms
= 2,500,000 ohms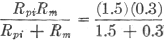

 Example: In the case of Fig. 9,
it is assumed that the grid is to be fed a square-wave pulse. Compare the plate
voltage when the tube is conducting a current of 15 ma. with the effective plate
voltage when the tube is idle and not drawing plate current. The plate resistance
is 10,000 ohms.
Example: In the case of Fig. 9,
it is assumed that the grid is to be fed a square-wave pulse. Compare the plate
voltage when the tube is conducting a current of 15 ma. with the effective plate
voltage when the tube is idle and not drawing plate current. The plate resistance
is 10,000 ohms. 
