Electric Space Ships, January 1951 Radio-Electronics

We've all heard of a Van de Graaff (Robert J.) electrostatic generator, but have you heard of a Wommelsdorf (Heinrich) generator (aka influence machine)? I hadn't prior to reading this article entitled "Electric Space Ships" from a 1951 issue of Radio-Electronics magazine. It took careful reading to get my head around the propulsion scheme detailed by Professor Hermann. At first I thought he was describing electric propulsion, but in fact his machine uses electrical energy generated from the sun to accelerate some form of liquid propellant via static electric charges. Therefore, this is not a system capable of indefinitely sustaining itself and its payload (which includes human inhabitants). In fact, the good professor proposes a geostationary refueling station above the Earth's equator which can be used to facilitate interplanetary exploration. It is a very interesting concept, but history shows the idea never got off the ground - so to speak. Here is Electric Space Ships Part 1.

The author's concept of a string of the electric spaceships on a flight through outer space. Because there is no gravity, the saucer-like ships can be made extremely light by earthly standards, although they cannot land on a planet.

In this second article of this series we shall discuss the details of a spaceship and its power plant - the energy source being the sun's rays. A large mirror concentrates solar heat on a specially constructed boiler. The vapor produced by the boiler drives a turbine, and the turbine in turn drives a special electric generator which provides both the propelling force and control for the ship.

The parabolic mirror 1 (see Fig. 1) reflects the sunlight on the boiler 2 which drives the dynamo. (Whether this should be a dynamo or an influence machine of the Wommelsdorf type depends on the behavior of the electrodes in outer space, a factor which cannot yet be predicted. My present feeling is that the Wommelsdorf machine is most suitable.)

The side of the boiler toward the mirror has a dark surface, while the side away from the mirror has a reflecting surface to reduce heat loss. The elements marked 3 are supporting rods. Like the mirror, these can be very lightly built (by earthly standards) because of the extraordinarily slight acceleration.

Fig. 1 - Drawing showing the essential parts of the solar-powered spaceship.

An exhaust pipe 4 leads the vapor in a spiral to the shady side of the mirror where the vapor condenses. Another pipe leads the condensed vapor back to the boiler. (I purposely avoid using the terms water and steam because other liquids are better suited for this machine.)

The two electrode couples are marked 5, and are of the type described in the first article. These can be rotated about axis 6. Since the mirror itself can be rotated about its own axis and the direction of this axis is that of the sun's rays, the recoil can be made to work in any direction in space. With the couples in the position shown, acceleration takes place in the direction of the arrow.

The slight acceleration which the recoil imparts to the apparatus is not enough to separate the vapor and the liquid from each other, and even this slight acceleration is available only after the boiler begins to function. Furthermore, the liquid can collect on the side facing the mirror only when the acceleration is toward the sun; otherwise the empty wall would be heated.

We can overcome this difficulty as well as gain other constructional advantages by allowing the entire cylindrical boiler to rotate about its own axis. Fig. 2 is a sketch of the boiler. Here 1 is the boiler and 2 is the working liquid which, because of the rapid rotation, collects on the boiler walls. The vapor collects in the middle of the boiler and flows to the turbine 3, which I have taken to be a two-stage machine. The guide vanes of the turbine are rigidly connected to the boiler wall.

The vapor then enters pipe 4 which leads it to the shadow of the mirror, where it is condensed, and the liquid returns through pipe 5. From 5 the liquid enters funnel 6 which is rigidly attached to the boiler and rotates with it. Centrifugal force then drives the liquid out of 6 and into the boiler through the tubes marked 7. Valves to prevent backflow of the liquid are marked 8.

The rotating vanes 10 of the turbine are mounted on shaft 9 which rotates within the boiler but in the opposite direction. The rotor runs at a hyper-critical velocity and therefore acts as its own counterbalance.

The source of current, here taken to be a Wommelsdorf machine (an improved influence machine invented in 1922), is indicated by 13, and 12 is a special coupling unit. The space within this machine is at the same pressure as the boiler for better efficiency and simpler construction.

Fig. 2 - A simplified cross-section of the vapor driven power plant proposed for the spaceship. The entire unit can be sealed against losses of the vapor.

The construction of the machine would be greatly simplified if the vapor within the boiler could flow freely in and out through 11, but this is not possible if water is used as the working fluid. The stator of the current generator 13 rotates in the same direction as the boiler, while the rotor turns with shaft 9. Slip rings are marked 14 and 14', while 15 and 15' are current collectors. The potential difference between these will be in the order of several thousand volts, but it is possible to insulate the rings very effectively in gravitationless space.

In Fig. 2 we also have 16, a casing which contains the whole machine. On the side toward the mirror is a window 17 which is permeable to both heat and light, and the rest of 16 is made of reflecting sheet metal. The outside of the boiler is black. The space between 1 and 16 or 17 is, of course, filled with vapor at the temperature of the boiler and at the same pressure as the exhaust vapor at 4.

No rotating parts of the machine pierce the outer wall at 4, 5, 16, or 17. The machine can therefore be effectively sealed off against losses of the working liquid - a most necessary measure.

c. Finally, the casing 16 together with all the other equipment, rods, mirror, electrodes, etc., can be brought to rotate about the boiler shaft.

In fact, because of the friction in bearings 18 and 19, the boiler must eventually impart its rotation to the rest of the device, while the turbine rotors 3 and the rotors of the current generator 13 absorb the opposite momentum.

I have therefore provided for another influence machine 20, whose stator is rigidly connected with the outer casing 16 while its rotor is similarly fastened to the shaft of the boiler. Depending on which direction the current flows through this machine, it will exert a turning moment on 16. Thus it is backed up, so to speak, on the boiler and accordingly turns the rest of the machine in the right position.

It would be advantageous to connect several such mirror engines with cables which, because of the small current, can be quite thin. The artist's drawing shows a string of such engines. In the foreground, to the right is the shelter or cabin for the space travelers. Ordinarily they occupy two chambers, connected by a long cable, which rotate about the common center of gravity. In this way the illusion of weight arises. Near the center of gravity is a pair of electrodes which draws its power from the mirror engines through the common cable. One can also imagine a switch point placed between the two electrodes to connect the influence machines either in parallel or in series.

We must now say something about the propulsive power and the efficiency of the electric spaceship.

The machines naturally perform better the stronger the sunlight. For example, in the vicinity of Venus they could accomplish twice as much as in the neighborhood of the earth. But of course the exact value of the solar constant outside the earth's atmosphere is unknown. Since the appropriate measurements are not available, I would guess it to be about 2.2 gram-calories per square centimeter per minute, and for our purpose this is close enough. Converted into the corresponding values for square meters and meter-kilograms per second, the radiation falling on each square meter is 156 mkg/sec. We can assume that the boiler can use about 30% of this energy, which might appear to be quite high, but we are justified in assuming a high operating efficiency here. The influence machine would, in turn, convert about 95% of this into electrical energy, which, expressed in m-g/sec per square meter of the mirror surface, comes to

We can assume that without the fuel the apparatus weighs 400 grams per m². This appears to be very small, but because of the slight requirements which they must meet from a statics point of view, these machines do not need to be heavier. Naturally one can take as much propellant for such a machine as is desired.

The first problem which could be solved - which, incidentally could be solved with liquid propellants only at great, unnecessary expense - would be the construction of a station circulating about the earth at a distance of 42,100 km (about 25,300 miles) above the center of the earth or 35,700 km (about 21,420 miles) above its surface at a velocity of 1,723 m/sec (3,850 miles an hour). Such a station would always hover above the same point on the equator if its orbit were in the equatorial plane. Otherwise it would describe a figure eight, as seen from the earth, which would bring it over the same point of the equator twice a day.

Such a station would be extremely valuable for television as well as for many other, particularly military, uses.

The electric spaceship could also carry out interplanetary flights, and indeed, this could be done in several months, whereas it has been estimated that such voyages would have to be reckoned in terms of years if liquid propellants alone were used (cf. Hohmann: Die Erreichbarkeit der Himmel-skorper. [The Attainability of the Celestial Bodies]).

In any case this spaceship could not land on one of the larger celestial bodies - it is far too weakly constructed for that. For this purpose it would have to carry a space boat which would be powered with atomic energy or with liquid propellant, while the spaceship itself circled about the celestial body like an observer's station without further expenditure of fuel.

Besides these two possibilities - powering the space boat with fuel or with atomic energy - there is a third, at least for visiting celestial bodies which have no atmosphere, like the moon.

Corpuscular radiations contain very little matter. At high potentials they have only a very slight impact force, but they do have a high energy content. We can also assume that, given high enough velocities, the particles would travel a great distance in parallel paths.

Equally charged mass-particles repel each other, but currents traveling in the same direction attract each other. Moving-charged particles are electric currents. If we can impart a high enough velocity to streams of particles, it is reasonable to assume that the particles would no longer tend to fly apart. Of course this will have to be tested first on a station in cosmic space, for I would not care to extrapolate blindly the results obtained from Geissler tubes and cyclotron streams to the corpuscular streams from an electric spaceship. I assume that this would certainly succeed with electron streams, but whether it would work with positive rays is something I do not care to affirm.

The spaceboat could receive in a Faraday cage the corpuscles streaming toward it and with this help generate an electric wind whose reaction is sufficient for landing on Mercury, the moon, or Ceres. Since it is also much lighter than the spaceship and does not have to carry the latter's machinery with it, its acceleration can be much greater and its construction heavier and more compact.

From our estimated solar constant we might expect a kinetic energy of 8.8 to 14.6 mkg/sec m². If we wish to give the propellant a velocity of 10 km/sec, then 1 gram of the latter contains kinetic energy equal to 5,100 m/kg. It therefore follows that at a distance of 150 million kilometers from the sun, energy can be radiated at a rate of 8.8:5100 = 1.7 mgr/sec to 14.6:5,100 = 3 mgr/sec per second per square meter of the mirror surface.

If the electric spaceship carries 2 kg (1 kg = 2.2 lbs.) of matter per square meter of mirror surface, this would suffice for a period of acceleration of from 670,000 sec. or 8 days to 1,170,000 sec. or 14 days.

I have added the subscript i to v because the spaceship would attain this velocity only in gravitationless space. If it started out from a station rotating about the earth, it would have to exceed the velocity of the station. In so doing, the original circular orbit (in the sense indicated by the Keplerian laws of planetary motion) would first have to pass over to an elliptical orbit; however, the ellipse would not be completed, but in each instant it would develop into an ever wider ellipse, so long as the machine operates.

Accordingly the spaceship ascends in a spiral path, the differential equation of which cannot be integrated in a closed expression. And its velocity actually diminishes in the process at the same time that its total energy, because of the increment of potential energy, increases. Thus v_i indicates only the so-called ideal velocity. I would also like to point out that of this 17 km/sec only about one-half or 9,000 m/sec can be used for propulsion, while the remainder must be used to check the velocity in the vicinity of the goal.

The following formulas refer to fuel consumption, increase of velocity, and the duration of the acceleration:

where m is the mass of the electric spaceship, m₀ its initial mass, t the time. and m' the quantity of fuel or propellant expelled during each second. Obviously the spaceship expels the same quantity of propellant in each second, since its distance from the sun does not change so rapidly, and, to save time, the machines are allowed to run at the highest number of revolutions per minute so long as they must operate. From (1) and the equation for velocity increase (see Part I) we obtain the increase in velocity between the times t₁ and t₂:

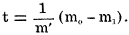
It is obvious that all the energy derived from the source of current will not be used for the acceleration of the propellant. A large part of it is lost in the charging processes going on. Moreover, not all the corpuscles fly off at the same speed, so that here too a certain amount of energy is lost.

It would take us too far afield, and would also be pointless in view of the uncertainty of the estimates referred to above, to describe exactly how I arrived at the values used. But I estimate that the kinetic energy of the expelled particles constitutes about one-fifth to one-third of the electrical energy supplied. While this may seem a rather low efficiency, it is no problem as we have an unlimited energy source from the sun.

With an exhaust or repulsion velocity of 20 km/sec, V₁ would therefore be twice as great, i.e., 35,836 m/sec; but from 32 to 56 days would then be required for the approach and slowing down for landing.

Electric Space Ships - Part 2January 1951 Radio-Electronics

Electric Space Ships - Part 2
January 1951 Radio-Electronics