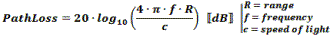|
 As a transmitted
signal traverses the atmosphere its voltage potential decreases at a rate proportional to the distance traveled (3 dB per distance doubling) and the
power level decreases at a rate proportional to the square of the distance traveled
(6 dB per distance doubling). Note that path loss is equally affected by frequency,
so doubling the frequency also results in 6 dB path loss per distance doubling.
The formula used by
RF Workbench
accounts for only the diminishing voltage without accounting for
absorption
or dispersion by the atmosphere. Both a 1-Way and a 2-Way Path Loss Calculator are
included in
Espresso Engineering Workbook™ for FREE.
These equations are valid when the distance is greater than about a wavelength.
At distances less than that the electric field is transitioning between near field
and far field where, physical factors like antenna dimensions dominate and force
the use of electromagnetic field equations.
Note: When using these formulas, be sure to keep dimensional
units consistent; i.e., do not mix kHz with MHz, mm with inches, etc. It is safer
to use base units (e.g., Hz, m) for calculation, then convert result to desired
units.
1-Way Power Path Loss Equations
(double for 2-way radar signal loss)
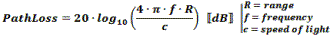
Friis Equation
The Friis Equation (H.T. Friis, 1946) gives a more complete accounting for all
the factors from the transmitter to the receiver:
Information in the transmitted signal is seldom concentrated at a single frequency,
so the path loss will actually be different for every frequency component in the
signal. Fortunately, the ratio of the bandwidth to center frequency is usually small
enough to not matter. Still, a signal that is transmitted with a constant power
across some bandwidth will appear at the receiver with a power slope that decreases
at the upper end of the band.
Free Space 1-Way Path Loss (km)
Free Space 1-Way Path Loss (miles)
Here is information on propagation time,
radar equation and
Doppler.
|
Most RF engineers are familiar with the
Friis equation, which predicts the received power level.
Harald T. Friis, born in Naestved, Denmark, is its namesake.
Just as with "Fresnel" (Fresnel zone),
many people do not know the proper pronunciation of "Friis" - I did not. So, I asked
a native Dane, Jørgen Jakob Friis, how he pronounces the name. He responded with
actual audio files of him speaking H.T. Friis' name and hometown.
 Friis |
Harald T. Friis
|
Naestved
|
|
