|
January 1964 Radio-Electronics
 [Table of Contents] [Table of Contents]
Wax nostalgic about and learn from the history of early electronics.
See articles from Radio-Electronics,
published 1930-1988. All copyrights hereby acknowledged.
|
The decibel is not a
concept unique to electronics - power, volts, current - although it is
undoubtedly most often used there. Probably the next most often used realm for
decibels is with sound (audio), which is the subject of this 1964
Radio-Electronics magazine article. The decibel, abbreviated nowadays
as "dB" ("db" in the article's era) is nothing more than a logarithmic
representation of a dimensionless ratio of increase (positive dB) or decrease
(negative dB). As the numerical "deci" implies, a decibel is one tenth of a bel
("B," named in honor of Alexander Graham Bell). It can be applied to any
numerical magnitude comparison. Although not normally done, a decibel ratio
could be applied to dissimilar units; for instance, a ratio of 100 apples to 50
oranges is 3 dB. Conversely, a ratio of 50 apples to 100 oranges is -3 dB.
Mr. King provides the gory mathematical details.
What Is a Decibel?
- Logs and db's for people who aren't full-time mathematicians.
 By David A. King By David A. King
What is a decibel? Is it really a measure of sound? Has it any use, other than
to confuse the listener (as well as impress him a little bit)? Let us take these
questions one at a time. (Some of you know the answers, but join us any-way. You
might pick up something new.)
1. The decibel is a convenient measure of a power ratio.
2. It is a measure of relative sound intensity, but it is a great deal more than
that!
3. Yes, the db has many uses other than to toss off to impress a listener when
discussing your hi-fi system.
Let's take a look at how the decibel got started. Long before the electronics
art was born, telephone engineers discovered that the human ear's perception of
sound did not depend directly on the power received. The ear has a built-in regulating
device that adjusts it to sounds of different intensities. It can make the sound
of a single cricket seem almost deafening - yet that same ear, a few moments later,
may be able to experience a minimum of pain in a boiler factory.

" ... a built-in regulating device that ... can make the sound of a cricket almost
deafening, yet ... a few minutes later, experience minimum pain in a boiler factory."

Fig. 1 - How can we express the gain of an amplifier delivering 20 watts output
for 1 volt input? See text.

Fig. 2 - Having a reference level lets us express levels in db - or, more properly,
dbm.
But that ability to adjust, which makes the ear so useful to us, makes its response
to varying power levels a long way from linear. Early experimenters established
that this response was logarithmic.
Whoa, there! We've used a new term here, with no explanation. So before we continue
with db, let's take a moment to catch up! (Advanced math experts will skip this
part!) What is a logarithm? Well, when we want to express a large number, we often
use a power of 10 rather than a string of zeros. Thus 1,000,000 can be expressed
as 106 (read "10 to the 6th"). This means that 10 X 10, 6 times over,
is 1,000,000. Note that 1,000,000 has six zeros. Another way of thinking of a power
of 10 is as 1 followed by the number of zeros indicated by the exponent - the little
number above and to the right of the 10.
A logarithm is a special kind of exponent: 101 is 10 itself; 102
is 100, but there are quite a few numbers between 1 and 10 and between 10 and 100
that we might be interested in using! Logically, if 101 is 10 and 102
is 100, 10 raised to 1-plus-some-decimal-fraction should equal some number between
10 and 100. This is exactly true. Logarithm tables give us just these decimal fractions,
leaving the assignment of the whole number portion of the logarithm up to our common
sense. Thus, 101 is 10, and 1 is thus the log of 10. 101.30103
is 20, and therefore 1.30103 is the log of 20 (as far as the fifth place).
These logarithms are called "base-10" or common logarithms, because they are
powers to which 10 must be raised to get the desired number. (There are other types
of logarithms which will not concern us at the moment.) 102 equals 100;
therefore the logarithm to the base 10 (written log10) of 100 is 2. The
little 2 in the exponent has been written full size as a regular number. 102.30103
equals 200, therefore log10 of 200 is 2.30103. The log is the power to
which the base (10 in this case) must be raised to equal the desired number.
Note that the characteristic or whole-number portion of the log of 200 is the
same as that for 100. Any figure with three places to the left of the decimal point
has a characteristic of 2. [103 is 1,000 (four places). Therefore, the
log of 1,000 is 3.] An easy rule for figuring the characteristic is that it is one
less than the total number of digits in any whole number (one less than the total
number of digits to the left of the decimal place). Thus the characteristic of all
numbers between 1 and 9 is zero. (10 to the zero power is 1). Thus, 100.30108
equals 2, or 0.30103 is the log of 2, etc.
What then determines whether the log is for 1 db for some other number? The
fractional part, or mantissa!
Roll Your Own Logs
How can we find the logarithm of any number? Let's practice with one or two numbers.
First, 3.445. Since the number of digits to the left of the decimal is 1, the characteristic
is 1 - 1, or zero. Now, refer to a logarithm table (you may have to borrow a friend's
math book for this if you haven't one of your own) and look up the succession of
digits 3445. (Disregard the decimal. It merely helps us to determine the characteristic.)
The mantissa of our log is 53719 (in a. five-place table). Putting the whole log
together, we have 0.53719. That's the correct logarithm. Looking at it another way,
that means that 10 is raised to the 0.53719 power, to equal 3.445 (100.53719
equals 3.445). The common log of 34.45 is 1.53719; that of 344.5 is 2.53719, and
of 3445.0 is 3.53719. See?
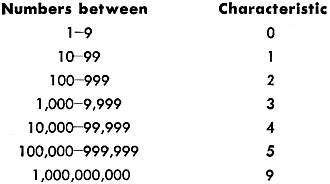
Table of Characteristics - Characteristic is consistently one less than total number of digits to left of
decimal point. This is true even when number contains decimal fraction. Example:
Characteristic of 324.9978 is the same as that of 324: namely, 2.
Try to find the common logarithms of a few more numbers. Logs are like any other
tool - very useful once mastered, but they require some practice for their mastery.
But What About the Decibel?
The formula used in decibel calculations is db = 10 log10 P2/P1. P
stands for power. In other words, a decibel is 10 times the common logarithm of
the ratio of two powers. Let's apply that formula. We have an amplifier with an
output of 20 watts, produced when it gets a 1-volt rms signal across its input (Fig.
1). The input resistance (probably the grid resistor of the first tube) is 1 megohm.
What's the gain of the amplifier?
So we won't start adding peaches and plums, let's convert the input to power.
(We could convert the output to volts across a known load resistor, but then our
formula would be a little different.) 1 volt across 1 megohm is 0.000001 watt (or
10-6 in the much neater power-of-10 notation). So now we have 20 watts
output (P2) and 1 microwatt of input (P1). Applying our formula, we get
10 log (20/0.000001)
or 10 times the log of 20,000,000.
Now remember: the characteristic of the log is 1 less than the number of digits,
so it is 1 less than 8, or 7. The mantissa is the same as that of 2, which we know
already is approximately 0.3010. The log of 20,000,000 then is 7.3010. And 10 times
that log is 73.010, or, more practically, 73 db. To find how much gain you need
to bring the known input to a desired power output level, just reverse the process.
Suppose we have a mike rated at -75 dbm, and want to use it with a preamp-amplifier
system to produce 15 watts (Fig. 2). How much power gain must the system have? Microphones
are almost universally rated against a reference level of 1 milliwatt. To distinguish
"db with respect to 1 mw" from ordinary decibels, we use the term "dbm".
A simple way to handle this problem is to refer to the output of the amplifier
system also in terms of 1 mw (0.001 w). Then we add our microphone and amplifier
dbm figures to get the total gain (and in db now, not dbm).
dbm = 10 log 15 watts/0.001 watt
= 10 log 15,000
= 10 X 4.1761
= 41.761
- or almost 42 dbm (42 db referred to 1 mw). Now we know that it takes 75 db
gain to raise the microphone output to the reference level, and another 42 db to
get 15 watts of audio power above that level. The total gain, then, is:
75 + 42 = 117 db.
Floating Decibels?
You can well ask, "If db must be referred to a reference level to have any meaning,
what use is a db which is not referred to any base? For instance, a pad is said
to have a loss of 10 db, or an amplifier gain of 60 db?" The answer is that both
statements refer to ratio. In other words, the pad will deliver one-tenth of the
power fed in at its input terminal; the amplifier will multiply the power fed to
its input terminals by a million.
Voltage and Current db
But, you say, you are more used to hearing the output of a preamp, for example,
expressed in volts, not milliwatts! Decibels are just as easy to use with voltage
ratios. And, since current gain and loss crop up frequently in transistor circuits,
we need current db as well. There is one difference in the formula for voltage or
current decibels and that for power. You remember that power is proportional to
the square of the voltage or current of the circuit. (Remember, E2/R
= P, and I2R = P?)
To square a number (raise it to the power 2) we multiply its log by 2 (102
= 100). So instead of the formula 10 log P2/P1, we have 20 log E2/E1, or 20 log
I2/I1, where E and I are voltage and current values. Now we're equipped to handle
db calculations in terms of power, voltage or current, and not only in audio or
acoustics, but anywhere we want to express the ratio of two powers, voltages or
currents: RF gain, signal-to-noise ratio, tuned circuit selectivity, FM capture
ratio. All these and others are very conveniently expressed in decibels.
Posted January 23, 2023
|







 By David A. King
By David A. King 



