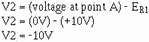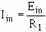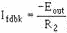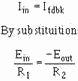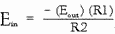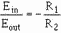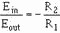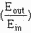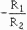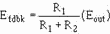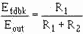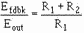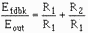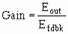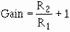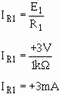Module 8 - Introduction to Amplifiers
Pages i,
1-1,
1-11,
1-21,
1-31,
2-1,
2-11,
2-21,
2-31,
3-1,
3-11,
3-21,
3-31,
3-41,
3-51,
3-61,
AI-1,
Index
| - |
Matter, Energy,
and Direct Current |
| - |
Alternating Current and Transformers |
| - |
Circuit Protection, Control, and Measurement |
| - |
Electrical Conductors, Wiring Techniques,
and Schematic Reading |
| - |
Generators and Motors |
| - |
Electronic Emission, Tubes, and Power Supplies |
| - |
Solid-State Devices and Power Supplies |
| - |
Amplifiers |
| - |
Wave-Generation and Wave-Shaping Circuits |
| - |
Wave Propagation, Transmission Lines, and
Antennas |
| - |
Microwave Principles |
| - |
Modulation Principles |
| - |
Introduction to Number Systems and Logic Circuits |
| - |
- Introduction to Microelectronics |
| - |
Principles of Synchros, Servos, and Gyros |
| - |
Introduction to Test Equipment |
| - |
Radio-Frequency Communications Principles |
| - |
Radar Principles |
| - |
The Technician's Handbook, Master Glossary |
| - |
Test Methods and Practices |
| - |
Introduction to Digital Computers |
| - |
Magnetic Recording |
| - |
Introduction to Fiber Optics |
| Note: Navy Electricity and Electronics Training
Series (NEETS) content is U.S. Navy property in the public domain. |
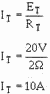
The voltage drop across R1 can be computed:

The voltage at point a would be equal to the voltage of V1 minus the voltage
drop of R1.

To check this result, compute the voltage drop across R2 and subtract this from
the voltage at point
A. The result should be the voltage of V2.

It is not necessary that the voltage supplies be equal to create a point of virtual
ground. In view (B) V1 supplies +1 volt to the circuit while V2 supplies -10 volts.
The total difference in potential is 11 volts. The total resistance of this circuit
(R1 + R2) is 11 ohms. The total current (IT) is 1 ampere. The voltage drop across
R1 (ER1 = R1 ´ IT) is 1 volt. The voltage drop across R2 (ER2
= R2 ´ I T) is 10 volts. The voltage at point a can be computed:

So point a is at virtual ground in this circuit also. To check the results, compute
the voltage at V2.
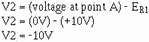
3-21
You can compute the values for view (C) and prove that point a in that circuit
is also at virtual ground.
The whole point is that the inverting input to the operational amplifier shown
in figure 3-13 is at virtual ground since it is at 0 volts (for all practical purposes).
Because the inverting input is at 0 volts, there will be no current (for all practical
purposes) flowing into the operational amplifier from the connection point of R1
and R2.
Given these conditions, the characteristics of this circuit are determined almost
entirely by the values of R1 and R2. Figure 3-15 should help show how the values
of R1 and R2 determine the circuit characteristics.

Figure 3-15. - Current flow in the operational circuit.
Note: It should be stressed at this point that for purpose of explanation the
operational amplifier is a theoretically perfect amplifier. In actual practice we
are dealing with less than perfect. In the practical operational amplifier there
will be a slight input current with a resultant power loss. This small signal can
be measured at the theoretical point of virtual ground. This does not indicate faulty
operation.
The input signal causes current to flow through R1. (Only the positive half cycle
of the input signal
is shown and will be discussed.) Since the voltage at the inverting input of
the operational amplifier is at 0 volts, the input current (Iin) is computed by:
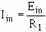
The output signal (which is opposite in phase to the input signal) causes a feedback
current (Ifdbk) to flow through R2. The left-hand side of R2 is at 0 volts (point
A) and the right-hand side is at Eout. Therefore, the feedback current is computed
by:
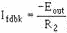
(The minus sign indicates that Eout is 180 degrees out of phase with Ein
and should not be confused with output polarity.)
3-22
Since no current flows into or out of the inverting input of the operational
amplifier, any current reaching point a from R1 must flow out of point a through
R2. Therefore, the input current (Iin) and the feedback current (Ifdbk) must be
equal. Now we can develop a mathematical relationship between the input and output
signals and R1 and R2.
Mathematically:
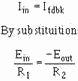
If you multiply both sides of the equation by R1:
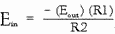
If you divide both sides of the equation by Eout:
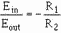
By inverting both sides of the equation:
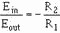
You should recall that the voltage gain of a stage is defined as the output voltage
divided by the input voltage:
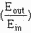
Therefore, the voltage gain of the inverting configuration of the operational
amplifier is expressed by the equation:
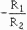
(As stated earlier, the minus sign indicates that the output signal is 180 degrees
out of phase with the input signal.)
Noninverting Configuration
Figure 3-16 shows a noninverting configuration using an operational amplifier.
The input signal (Ein) is applied directly to the noninverting (+) input of the
operational amplifier. Feedback is provided by
3-23
coupling part of the output signal (Eout) back to the inverting (-) input of
the operational amplifier. R1 and R2 act as voltage divider that allows only a part
of the output signal to be applied as feedback (Efdbk).
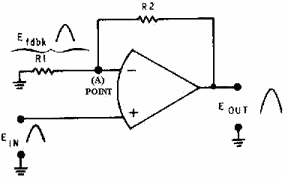
Figure 3-16. - Noninverting configuration.
Notice that the input signal, output signal, and feedback signal are all in phase.
(Only the positive alternation of the signal is shown.) It may appear as if the
feedback is regenerative (positive) because the feedback and input signals are in
phase. The feedback is, in reality, degenerative (negative) because the input signals
is applied to the noninverting input and the feedback signal is applied to the inverting
input, (Remember, that the operational amplifier will react to the difference between
the two inputs.)
Just as in the inverting configuration, the feedback signal is equal to the input
signal (for all practical purposes). This time, however, the feedback signal is
in phase with the input signal.
Therefore:

Given this condition, you can calculate the gain of the stage in terms of the
resistors (R1 and R2). The gain of the stage is defined as:

The feedback signal (Efdbk) can be shown in terms of the output signal (Eout)
and the voltage divider (R1 and R2). The voltage divider has the output signal on
one end and ground (0 volts) on the other end. The feedback signal is that part
of the output signal developed by R1 (at point A). Another way to look at it is
that the feedback signal is the amount of output signal left (at point A) after
part of the output signal
3-24
has been dropped by R2. In either case, the feedback signal (Efdbk) is the ratio
of R1 to the entire voltage divider (R1 + R2) multiplied by the output signal (Eout).
Mathematically, the relationship of the output signal, feedback signal, and voltage
divider is:
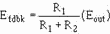
if you divide both sides of the equation by Eout:
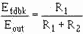
By inverting both sides of the equation:
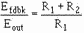
Separating the right-hand side:
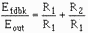
Remember:
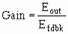
Therefore, by substitution:
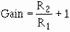
You can now see that the gain of the noninverting configuration is determined
by the resistors. The formula is different from the one used for the inverting configuration,
but the gain is still determined by the values of R1 and R2.
Bandwidth Limitations
As with most amplifiers, the gain of an operational amplifier varies with frequency.
The specification sheets for operational amplifiers will usually state the open-loop
(no feedback) gain for d.c. (or 0 hertz). At higher frequencies, the gain is much
lower. In fact, for an operational amplifier, the gain decreases quite rapidly as
frequency increases.
Figure 3-17 shows the open-loop (no feedback) frequency-response curve for a
typical operational amplifier. As you should remember, bandwidth is measured to
the half-power points of a frequency- response curve. The frequency-response curve
shows that the bandwidth is only 10 hertz with this
3-25
configuration. The UNITY Gain Point, where the signal out will have the same
amplitude as the signal in (the point at which the gain of the amplifier is 1),
is 1 megahertz for the amplifier. As you can see, the frequency response of this
amplifier drops off quite rapidly.
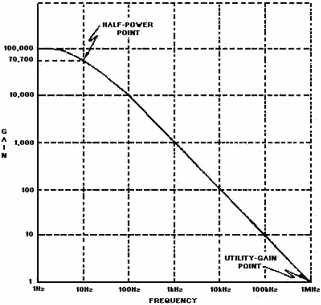
Figure 3-17. - Open-loop frequency-response curve.
Figure 3-17 is the open-loop frequency-response curve. You have been told that
most operational amplifiers are used in a closed-loop configuration. When you look
at the frequency-response curve for a closed-loop configuration, one of the most
interesting and important aspects of the operational amplifier becomes apparent:
The use of degenerative feedback increases the bandwidth of an operational amplifier
circuit.
This phenomenon is another example of the difference between the operational
amplifier itself and the operational-amplifier circuit (which includes the components
in addition to the operational amplifier). You should also be able to see that the
external resistors not only affect the gain of the circuit, but the bandwidth as
well.
You might wonder exactly how the gain and bandwidth of a closed-loop, operational-amplifier
circuit are related. Figure 3-18 should help to show you the relationship. The frequency-response
curve shown in figure 3-18 is for a circuit in which degenerative feedback has been used to decrease the circuit gain to 100 (from 100,000 for the operational amplifier).
Notice that the half-power point of this curve is just slightly above 10 kilohertz.
3-26
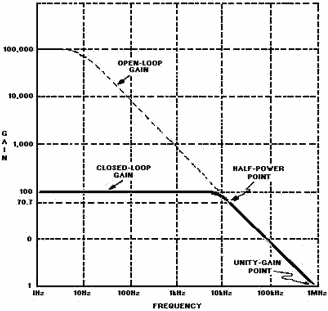
Figure 3-18. - Closed-loop frequency-response curve for gain of 100.
Now look at figure 3-19. In this case, more feedback has been used to decrease
the gain of the circuit to 10. Now the bandwidth of the circuit is extended to about
100 kilohertz.
3-27
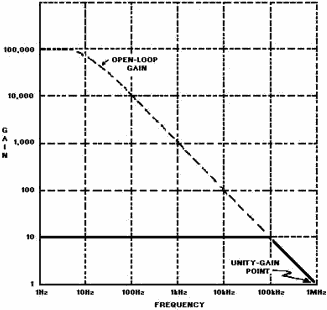
Figure 3-19. - Closed-loop frequency-response curve for gain of 10.
The relationship between circuit gain and bandwidth in an operational-amplifier
circuit can be expressed by the Gain-Bandwidth Product (Gain ´ Bandwidth = UNITY
Gain Point). In other words, for operational-amplifier circuits, the gain times
the bandwidth for one configuration of an operational amplifier will equal the gain
times the bandwidth for any other configuration of the same operational amplifier.
In other words, when the gain of an operational-amplifier circuit is changed (by
changing the value of feedback or input resistors), the bandwidth also changes.
But the gain times the bandwidth of the first configuration will equal the gain
times the bandwidth of the second configuration. The following example should help
you to understand this concept.
The frequency-response curves shown in figures 3-17, 3-18, and 3-19 have a gain-bandwidth
product of 1,000,000. In figure 3-17, the gain is 100,000 and the bandwidth is 10
hertz. The gain-bandwidth product is 100,000 times 10 (Hz), or 1,000,000. In figure
3-18, the gain has been reduced to 100 and the bandwidth increases to 10 kilohertz.
The gain-bandwidth product is 100 times 10,000 (Hz) which is also equal to 1,000,000.
In figure 3-19 the gain has been reduced to 10 and the bandwidth is 100 kilohertz.
The gain-bandwidth product is 10 times 100,000 (Hz), which is 1,000,000. If the
gain were reduced to 1, the bandwidth would be 1 megahertz (which is shown on the
frequency-response curve as the unity-gain point) and the gain-bandwidth product
would still be 1,000,000.
Q-19. What does the term "closed-loop" mean in the closed-loop configuration
of an operational amplifier?
In answering Q20, Q21, and Q23, select the correct response from the choices
given in the parentheses.
3-28
Q-20. In a closed-loop configuration the output signal is determined
by (the input signal, the feedback signal, both).
Q-21. In the inverting configuration, the input signal is applied
to the (a) (inverting, noninverting) input and the feedback signal is applied to
the (b) (inverting, noninverting) input.
Q-22. In the inverting configuration, what is the voltage (for all
practical purposes) at the inverting input to the operational amplifier if the input
signal is a 1-volt, peak-to-peak sine wave?
Q-23. In the inverting configuration when the noninverting input
is grounded, the inverting input is at (signal, virtual) ground.
Q-24. In a circuit such as that shown in figure 3-15, if R1 has a
value of 100 ohms and R2 has a value of 1 kilohm and the input signal is at a value
of + 5 millivolts, what is the value of the output signal?
Q-25. If the unity-gain point of the operational amplifier used in
question 24 is 500 kilohertz, what is the bandwidth of the circuit?
Q-26. In a circuit such as that shown in figure 3-16, if R1 has a
value of 50 ohms and R2 has a value of 250 ohms and the input signal has a value
of +10 millivolts, what is the value of the output signal?
Q-27. If the open-loop gain of the operational amplifier used in
question 26 is 200,000 and the open- loop bandwidth is 30 hertz, what is the closed
loop bandwidth of the circuit?
Applications of Operational Amplifiers
Operational amplifiers are used in so many different ways that it is not possible
to describe all of the applications. Entire books have been written on the subject
of operational amplifiers. Some books are devoted entirely to the applications of
operational amplifiers and are not concerned with the theory of operation or other
circuits at all. This module, as introductory material on operational amplifiers,
will show you only two common applications of the operational amplifier: the summing
amplifier and the difference amplifier. For ease of explanation the circuits shown
for these applications will be explained with d.c. inputs and outputs, but the circuit
will work as well with a.c. signals.
Summing Amplifier (Adder)
Figure 3-20 is the schematic of a two-input adder which uses an operational amplifier.
The output level is determined by adding the input signals together (although the
output signal will be of opposite polarity compared to the sum of the input signals).
3-29
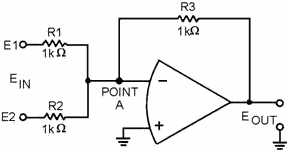
Figure 3-20. - Two-input adder.
If the signal on input number one (E1) is +3 volts and the signal on input number
two (E2) is +4 volts, the output signal (Eout) should be -7 volts [(+3 V) + (+4
V) = +7 V and change the polarity to get -7 V].
With +3 volts at E1 and 0 volts at point a (which is at virtual ground), the
current through R1 must be 3 milliamperes.
Mathematically:
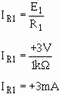
(The + sign indicates a current flow from right to left.)
By the same sort of calculation, with +4 volts at E2 and 0 volts at point a the
current through R2 must be 4 milliamps.
This means that a total of 7 milliamps is flowing from point a through R1 and
R2. If 7 milliamps is flowing from point A, then 7 milliamps must be flowing into
point A. The 7 milliamps flowing into point a flows through R3 causing 7 volts to
be developed across R3. With point A at 0 volts and 7 volts developed across R3,
the voltage potential at Eout must be a -7 volts. Figure 3-21 shows these
voltages and currents.
3-30
|