| - |
Matter, Energy,
and Direct Current |
| - |
Alternating Current and Transformers |
| - |
Circuit Protection, Control, and Measurement |
| - |
Electrical Conductors, Wiring Techniques,
and Schematic Reading |
| - |
Generators and Motors |
| - |
Electronic Emission, Tubes, and Power Supplies |
| - |
Solid-State Devices and Power Supplies |
| - |
Amplifiers |
| - |
Wave-Generation and Wave-Shaping Circuits |
| - |
Wave Propagation, Transmission Lines, and
Antennas |
| - |
Microwave Principles |
| - |
Modulation Principles |
| - |
Introduction to Number Systems and Logic Circuits |
| - |
- Introduction to Microelectronics |
| - |
Principles of Synchros, Servos, and Gyros |
| - |
Introduction to Test Equipment |
| - |
Radio-Frequency Communications Principles |
| - |
Radar Principles |
| - |
The Technician's Handbook, Master Glossary |
| - |
Test Methods and Practices |
| - |
Introduction to Digital Computers |
| - |
Magnetic Recording |
| - |
Introduction to Fiber Optics |
| Note: Navy Electricity and Electronics Training
Series (NEETS) content is U.S. Navy property in the public domain. |
Module 11 - Microwave Principles Pages
i,
1-1, 1-11,
1-21,
1-31,
1-41,
1-51,
1-61,
2-1,
2-11, 2-21,
2-31,
2-41,
2-51,
2-61,
3-1,
3-11,
AI-1,
Index-1,
Assignment 1,
Assignment 2
...Pages 1-11 through 1-20
Waveguide Theory and Application

Figure 1-27A. - Different frequencies in a waveguide.
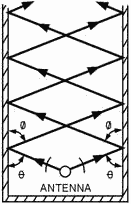
Figure 1-27B - Different frequencies in a waveguide.
The velocity of propagation of a wave along a waveguide is less than its velocity
through free space (speed of light). This lower velocity is caused by the zigzag
path taken by the wavefront. The forward-progress velocity of the wavefront in a
waveguide is called GROUP VELOCITY and is somewhat slower than the speed of light.
The group velocity of energy in a waveguide is determined by the reflection angle
of the wavefronts off the "b" walls. The reflection angle is determined by the frequency
of the input energy. This basic principle is illustrated in figures 1-28A, 1-28B,
and 1-28C. As frequency is decreased, the reflection angle decreases causing the
group velocity to decrease. The opposite is also true; increasing frequency increases
the group velocity.

Figure 1-28A - Reflection angle at various frequencies. Low Frequency.

Figure 1-28B - Reflection angle at various frequencies. MEDIUM
Frequency.

Figure 1-28C - Reflection angle at various frequencies. High
Frequency.
Q-14. What interaction causes energy to travel down a waveguide?
Q-15. What is indicated by the number of arrows (closeness of spacing) used to
represent an electric field?
Q-16. What primary condition must magnetic lines of force meet in order to exist?
Q-17. What happens to the H lines between the conductors of a coil when the conductors
are close together?
Q-18. For an electric field to exist at the surface of a conductor, the field
must have what angular relationship to the conductor?
Q-19. When a wavefront is radiated into a waveguide, what happens to the portions
of the wavefront that do not satisfy the boundary conditions?
Q-20. Assuming the wall of a waveguide is perfectly flat, what is the angular
relationship between the angle of incidence and the angle of reflection?
Q-21. What is the frequency called that produces angles of incidence and reflection
that are perpendicular to the waveguide walls?
Q-22. Compared to the velocity of propagation of waves in air, what is the velocity
of propagation of waves in waveguides?
Q-23. What term is used to identify the forward progress velocity of wavefronts
in a waveguide?
Waveguide Modes of Operation

Figure 1-29 - Half-sine E field distribution.

Figure 1-30 - Full-sine E field distribution.
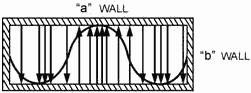
Figure 1-31 - One and one-half sine E field distribution.
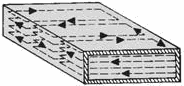
Figure 1-32 - Magnetic field caused by a half-sine E field.
The waveguide analyzed in the previous paragraphs yields an electric field configuration
known as the half-sine electric distribution. This configuration, called a Mode
of Operation, is shown in figure 1-29. Recall that the strength of the field is
indicated by the spacing of the lines; that is, the closer the lines, the stronger
the field. The regions of maximum voltage in this field move continuously down the
waveguide in a sine-wave pattern. To meet boundary conditions, the field must always
be zero at the "b" walls.
The half-sine field is only one of many field configurations, or modes, that
can exist in a rectangular waveguide. a full-sine field can also exist in a rectangular
waveguide because, as shown in figure 1-30, the field is zero at the "b" walls.
Similarly, a 1 1/2 sine-wave field can exist in a rectangular waveguide because
this field also meets the boundary conditions. As shown in figure 1-31, the field
is perpendicular to any conducting surface it touches and is zero along the "b"
walls.
The magnetic field in a rectangular waveguide is in the form of closed loops
parallel to the surface of the conductors. The strength of the magnetic field is
proportional to the electric field. Figure 1-32 illustrates the magnetic field pattern
associated with a half-sine electric field distribution. The magnitude of the magnetic
field varies in a sine-wave pattern down the center of the waveguide in "time phase"
with the electric field. TIME Phase means that the peak H lines and peak E lines
occur at the same instant in time, although not necessarily at the same point along
the length of the waveguide.
An electric field in a sine-wave pattern also exists down the center of a waveguide.
In figure 1-33, view (A), consider the two wavefronts, C and D. Assume that they
are positive at point 1 and negative at point 2. When the wavefronts cross at points
1 and 2, each field is at its maximum strength. At these points, the fields combine,
further increasing their strength. This action is continuous because each wave is
always followed by a replacement wave. Figure 1-33, view (B), illustrates the resultant
sine configuration of the electric field at the center of the waveguide. This configuration
is only one of the many field patterns that can exist in a waveguide. Each configuration
forms a separate mode of operation. The easiest mode to produce is called the DOMINANT
Mode. Other modes with different field configurations may occur accidentally or
may be caused deliberately.

Figure 1-33 - Crisscrossing wavefronts and the resultant E field.

Figure 1-34A - Waveguide operation in other than dominant mode.

Figure 1-34B - Waveguide operation in other than dominant mode.

Figure 1-36 - Dominant mode in a rectangular waveguide.

Figure 1-37 - Counting wavelengths in a circular waveguide.
The dominant mode is the most efficient mode. Waveguides are normally designed
so that only the dominant mode will be used. To operate in the dominant mode, a
waveguide must have an "a" (wide) dimension of at least one half-wavelength of the
frequency to be propagated. The "a" dimension of the waveguide must be kept near
the minimum allowable value to ensure that only the dominant mode will exist. In
practice, this dimension is usually 0.7 wavelength.
Of the possible modes of operation available for a given waveguide, the dominant
mode has the lowest cutoff frequency. The high-frequency limit of a rectangular
waveguide is a frequency at which its "a" dimension becomes large enough to allow
operation in a mode higher than that for which the waveguide has been designed.

Figure 1-35 - Dominant mode in a circular waveguide.
Waveguides may be designed to operate in a mode other than the dominant mode.
An example of a full-sine configuration mode is shown in figures 1-34A and 1-34B.
The "a" dimension of the waveguide in this figure is one wavelength long. You may
assume that the two-wire line is 1/4λ from one of the "b" walls, as shown in figure
1-34A. The remaining distance to the other "b" wall is 3/4λ. The three-quarter wavelength
section has the same high impedance as the quarter-wave section; therefore, the
two-wire line is properly insulated. The field configuration shows a complete sine-wave
pattern across the "a" dimension, as illustrated in figure 1-34B.
Circular waveguides are used in specific areas of radar and communications systems,
such as rotating joints used at the mechanical point where the antennas rotate.
Figure 1-35 illustrates the dominant mode of a circular waveguide. The cutoff wavelength
of a circular guide is 1.71 times the diameter of the waveguide. Since the "a" dimension
of a rectangular waveguide is approximately one half-wavelength at the cutoff frequency,
the diameter of an equivalent circular waveguide must be 2 ÷ 1.71, or approximately
1.17 times the "a" dimension of a rectangular waveguide.
Mode Numbering Systems
So far, only the most basic types of E and H field arrangements have been shown.
More complicated arrangements are often necessary to make possible coupling, isolation,
or other types of operation. The field arrangements of the various modes of operation
are divided into two categories: TRANSVERSE ELECTRIC (TE) and TRANSVERSE Magnetic
(TM).
In the transverse electric (TE) mode, the entire electric field is in the transverse
plane, which is perpendicular to the length of the waveguide (direction of energy
travel). Part of the magnetic field is parallel to the length axis.
In the transverse magnetic (TM) mode, the entire magnetic field is in the transverse
plane and has no portion parallel to the length axis.
Since there are several TE and TM modes, subscripts are used to complete the
description of the field pattern. In rectangular waveguides, the first subscript
indicates the number of half-wave patterns in the "a" dimension, and the second
subscript indicates the number of half-wave patterns in the "b" dimension.
The dominant mode for rectangular waveguides is shown in figure 1-36. It is designated
as the TE mode because the E fields are perpendicular to the "a" walls. The first
subscript is 1 since there is only one half-wave pattern across the "a" dimension.
There are no E-field patterns across the "b" dimension, so the second subscript
is 0. The complete mode description of the dominant mode in rectangular waveguides
is TE1,0. Subsequent descriptions of waveguide operation in this text will assume
the dominant (TE1,0) mode unless otherwise noted.
A similar system is used to identify the modes of circular waveguides. The general
classification of TE and TM is true for both circular and rectangular waveguides.
In circular waveguides the subscripts have a different meaning. The first subscript
indicates the number of full-wave patterns around the circumference of the waveguide.
The second subscript indicates the number of half-wave patterns across the diameter.
In the circular waveguide in figure 1-37, the E field is perpendicular to the
length of the waveguide with no E lines parallel to the direction of propagation.
Thus, it must be classified as operating in the TE mode. If you follow the E line
pattern in a counterclockwise direction starting at the top, the E lines go from
zero, through maximum positive (tail of arrows), back to zero, through maximum negative
(head of arrows), and then back to zero again. This is one full wave, so the first
subscript is 1. Along the diameter, the E lines go from zero through maximum and
back to zero, making a half-wave variation. The second subscript, therefore, is
also 1. TE 1,1 is the complete mode description of the dominant mode in circular
waveguides. Several modes are possible in both circular and rectangular waveguides.
Figure 1-38 illustrates several different modes that can be used to verify the mode
numbering system.

Figure 1-38. - Various modes of operation for rectangular and
circular waveguides.
Waveguide Input/Output Methods
A waveguide, as explained earlier in this chapter, operates differently from
an ordinary transmission line. Therefore, special devices must be used to put energy
into a waveguide at one end and remove it from the other end.
The three devices used to inject or remove energy from waveguides are PROBES,
LoopS, and SLOTS. Slots may also be called APERTURES or WINDOWS.
As previously discussed, when a small probe is inserted into a waveguide and
supplied with microwave energy, it acts as a quarter-wave antenna. Current flows
in the probe and sets up an E field such as the one shown in figure 1-39A. The E
lines detach themselves from the probe. When the probe is located at the point of
highest efficiency, the E lines set up an E field of considerable intensity.

Figure 1-39A - Probe coupling in a rectangular waveguide.

Figure 1-39B - Probe coupling in a rectangular waveguide.

Figure 1-39C - Probe coupling in a rectangular waveguide.

Figure 1-39D - Probe coupling in a rectangular waveguide.
The most efficient place to locate the probe is in the center of the "a" wall,
parallel to the "b" wall, and one quarter-wavelength from the shorted end of the
waveguide, as shown in figure 1-39B, and figure 1-39C. This is the point at which
the E field is maximum in the dominant mode. Therefore, energy transfer (coupling)
is maximum at this point. Note that the quarter-wavelength spacing is at the frequency
required to propagate the dominant mode.
In many applications a lesser degree of energy transfer, called loose coupling,
is desirable. The amount of energy transfer can be reduced by decreasing the length
of the probe, by moving it out of the center of the E field, or by shielding it.
Where the degree of coupling must be varied frequently, the probe is made retractable
so the length can be easily changed.
The size and shape of the probe determines its frequency, bandwidth, and power-handling
capability. As the diameter of a probe increases, the bandwidth increases. a probe
similar in shape to a door knob is capable of handling much higher power and a larger
bandwidth than a conventional probe. The greater power-handling capability is directly
related to the increased surface area. Two examples of broad-bandwidth probes are
illustrated in figure 1-39D. Removal of energy from a waveguide is simply a reversal
of the injection process using the same type of probe.
Another way of injecting energy into a waveguide is by setting up an H field
in the waveguide. This can be accomplished by inserting a small loop which carries
a high current into the waveguide, as shown in figure 1-40A. a magnetic field builds
up around the loop and expands to fit the waveguide, as shown in figure 1-40B. If
the frequency of the current in the loop is within the bandwidth of the waveguide,
energy will be transferred to the waveguide.
For the most efficient coupling to the waveguide, the loop is inserted at one
of several points where the magnetic field will be of greatest strength. Four of
those points are shown in figure 1-40C.
...Pages 1-31 through 1-40
Related Pages on RF Cafe
|














































